 |
| サイトマップ | |
|
 |
| サイトマップ | |
|
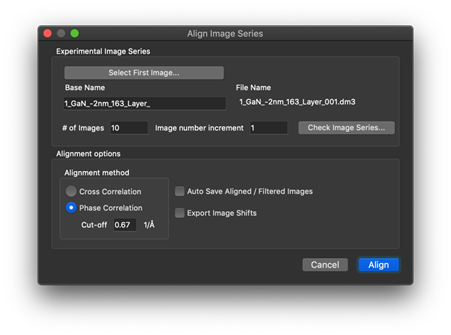
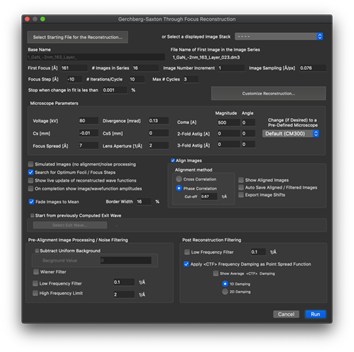
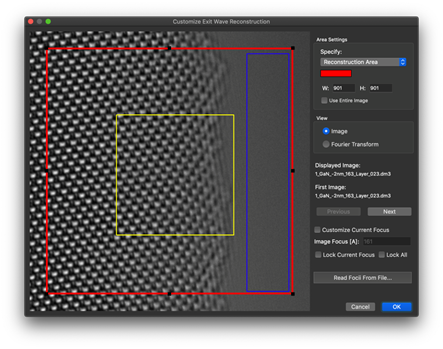
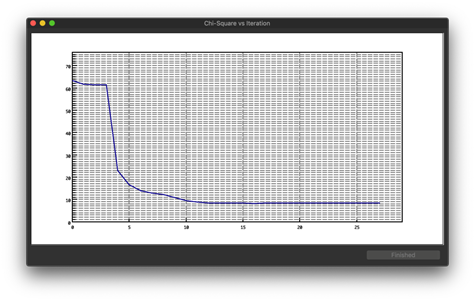
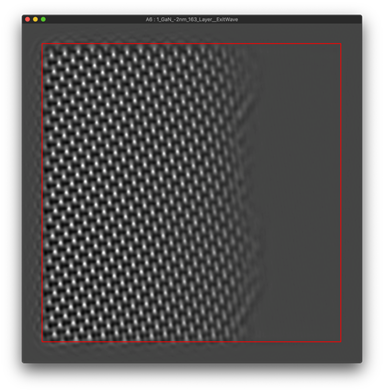
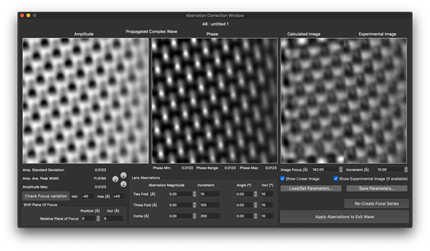
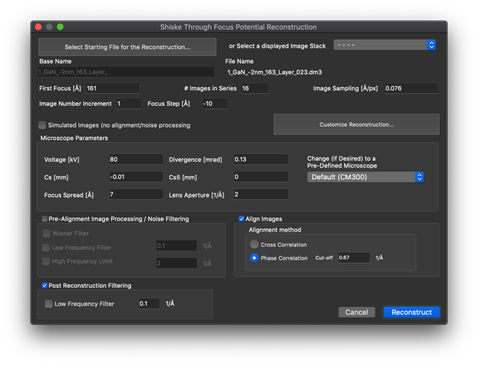
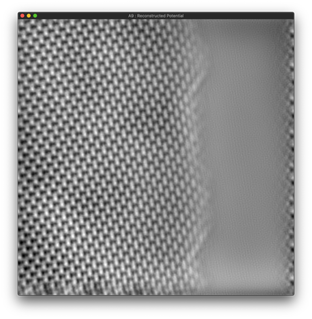
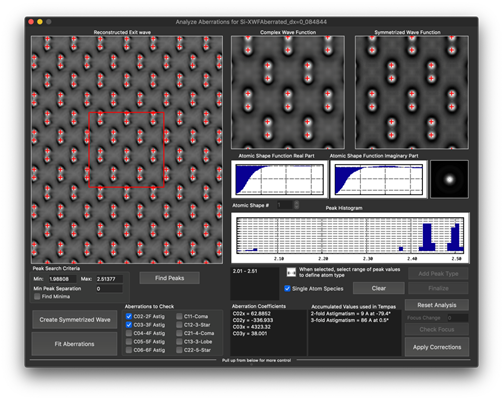
再構成ソフトウェアパッケージは、波面 (Exit Wave) 関数 (波動関数) と、焦点移動 (thru-focus-series) 、および、収差補正位相プレート、画像の整列、Shiske フィルタによるオブジェクトの復元をもとに標本ポテンシャルを求める各種アルゴリズムで構成されています。現在実装されている機能を以下に示します:
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
スクリプトの記述は、シミュレーション制御やイメージ処理を自動化することにより Tempas の機能を拡張する効果的な手段です。全く新しいアルゴリズムを作成してそれを試したり、様々なアイデアを実装することもできます。
Tempas のスクリプティング言語は、C, C++ によるプログラムの作成や、Digital Micrograph (copyright Gatan Inc.) 用のスクリプティング言語の経験のある方ならどなたでもすぐにご利用いただけます。このスクリプト言語には、Digital Micrograph のスクリプトを作成したことがある方のために、多くの DM スクリプトを殆ど修正なしで実行できる組み込み関数が用意されています。
最新のスクリプト言語のリファレンスマニュアルをダウンロードするには、こちらをクリックしてください。
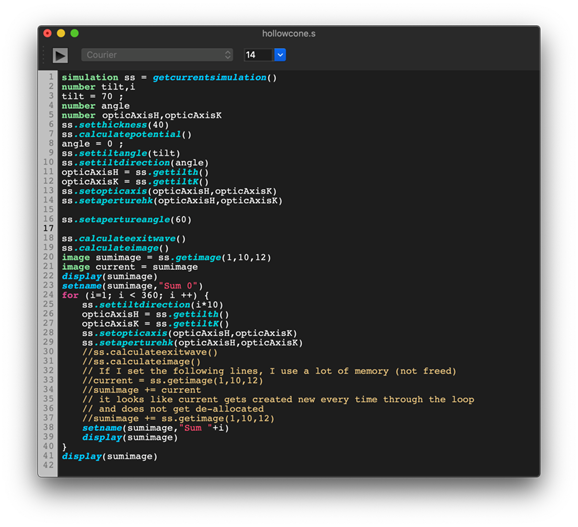 |
Image test = newimage(“test”,512,512) // Creates a float image of size 512 by 512 test = exp(-iradius*iradius/100) // Fills the image with a gaussian with sigma = 10 display(test) // Displays the image
Image test(512,512,exp(-iradius**2/100)) test.show() // Displays the image
Image test = newimage(“test”,512,512) // Creates a float image of size 512 by 512
test = sin(2*pi()*icol/8)+sin(2*pi()*irow/12)
// Fills the image with cross-fringes
// of period 8 and 12 pixels in x , y
display(test) // Displays the image
Image test(512,512,sin(2*pi*icol/8)+sin(2*pi*irow/12)) test.display() // Displays the image
/ Precession Tilt series
// This is summing over the power-spectrum of the exit wave function
// by spinning the beam in a circle. The beam tilt is theta (30 mrad).
// The increment in the azimuthal angle is dphi (6 degrees)
// A table of HKL values for different thicknesses is shown
// For illustration purposes, a precession image is also calculated
number theta = 30 // The tilt angle in mrad
number phi = 0 // Tilt angle (degrees) with respect to a-axis
number dphi = 6 // increments in tilt angle (degrees)
simulation sim = getsimulation() // Get the simulation
// We are making sure that everything has been calculated and is current
sim.calculateall()
image xw = sim.loadexitwave() // Declare and load the exit wave
image im = sim. loadimage() // Declare and load the image
image sumim = im ; sumim = 0 ; // Declare the sum for the images and zero
image sumps = xw ; sumps = 0 ; // Declare the sum for the powerspectrum
// and zero
Openlogwindow()
for(number thickness = 10; thickness <= 100; thickness += 10) {
sim.setthickness(thickness)
number i = 0 // declare and initialize our counter
for(phi = 0 ; phi < 360; phi += dphi) { // loop over the azimuthal angle
sim.settilt(theta,phi) // set the tilt of the specimen
// this is equivalent to the tilting the beam
sim.calculateexitwave() // Calculate the new exit wave
sim.calculateimage() // Calculate the new image
sumim += sim. loadimage() // Add the image to the sum
xw = sim. loadexitwave() // Load the exit wave
xw.fft() // Fourier transform to get the frequency
// complex coefficients
xw *= conjugate(xw) // Set the complex PowerSpectrum
// If we had used xw.ps() to get the
// power spectrum we would have had a real
// image in “real” space
sumps += xw // Add the powerspectrum to the sum
i++ // Keep track of the count
print("phi = "+phi) // Just to know where we are in the loop
}
sumim /= i // Divide by the number of terms in the sum
// Create a rectangular image of size 1024 by 1024 of sampling 0.1 Å (default)
image precessionImage = sim.createimage(sumim,1024)
precessionImage.setname("Image Precession")
precessionImage.show() // Show the summed images
sumps /= i // Divide by the number of terms in the sum
sumps.sqrt() // To compare with the Scattering factors
// Create a rectangular image of size 1024 by 1024 out to gMax = 4 1/Å
// with a convergence angle of 0.2 mrad
image precessionPS = sim.createfrequencyimage(sumps,1024,0.2,4)
precessionPS.setname("Power Spectrum Precession Thickness "+ sim.getthickness())
precessionPS.show() // Show the summed power spectrum
sumps.setname("thickness " + sim.getthickness())
sim.createhkltable(sumps)
}